Table 16.1 lEC 1000-3-2 Harmonic current limits, class Л

Odd harmonics

Even harmonics

16.6.2 IEEE/ANSI Standard 519

In 1993, the IEEE published a revised draft standard limiting the amplitudes of current harmonics, IEEE Guide fov Harmonic Control mid Reaclive Compensalioii of Sialic Power Converters. The harmonic limits are based on Ihe ratio of the fundamental component of the load current /; to the short circuit current at the point of common coupling (PCC) at the utility I. Stricter limits are imposed on large loads than on small loads. The limits are similar in magnitude to lEC 1000, and cover high voltage loads (of much higher power) not addressed by lEC 10(Ю. Enforcement of this standard is presently up to the local utility company.

The (xkl harnronic limits for general distribution systems at voltages of 120 V to 69 kV are listed in Table 16.2. The limits for even harmonics are 25% of the odd harmonic limits. Limits for general distribution systems at 69.001 kV to 161 kV are 50% of the values listed in Table 16.2. DC current components and half-wave rectifiers are not allowed.

It is the responsibility of the power consumer to meet these current harmonic standards. Standard lEEE-519 also specifies maximum allowable voltage harmonics, listed in Table 16.3. It is the responsibility of the utility, or power supplier, to meet these limits. Both total harmonic distortion and maximum individual harmonic magnitudes are limited.

Table 16.2 IEEE-519 Maxitnunt odd hartnonic current limits for general distribution systems, 120 V to 69 kV

liible 16.3 lEEE-S 19 Voltage distortion limits

BiBLIOfiKAPHY [1]

J. Arrillaga, D. Bradley, and P. Bodger, Power System Harnwnicx, New York: John Wiley & Sons, 1985.

[2] R. Smity and R. Stratford, Tower System Harmonics Effects from Adjustable-Speed Drives, IEEE Jramactions on htdiistrc Applications, Vol. IA-20, No. 4, pp. 912-411, July/August I9S4.

[3] A. Emanuel, Powers in Nonsinusoidal Situations-A Review of Definitions and Physical Meaning, IEEE Transactions on Power Delivery, Vol. 5, No. 3, pp. 1377-1389, July 1990.

[4] N. Mohan, T. UNDELAND, and W. ROBBINS, Power Electronics: Converters, Applications, and Design,

Second edition. New York: John Wiley & Sons, 1995.

[5] J. kassakian, M. SCHLECHT, and g. VERGE5E, Principles of Power Electronics. Massachusetts: Addison-Wesley, 1991.

[6] R. Gretsch, Harmonic Distortion ofthe Mains Voltage by Switched-Mode Power Supplies-Assessment of the Future Development and Possible Mitigation Measures, European Power Electronics Conference, Ш9 Record, pp. 1255-1260

[7] ШС 10003-2, First Edition, Commission Elecltotechnique Internationale, Geneva, 1995.

Problems 16.1

Passive rectifier circuit. In the passive rectifier circuit of Fig. 16.13, Lis very large, such that the inductor cuixent is essentially dc. All components are ideal.

230 Vrms 30 Hz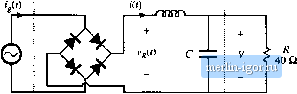

Fig. 16.13 Passlverectifiercircuitof Problem 16.1

(a) Delermine the dc output voltage, current, anJ power.

Power and Harmonics in Nottsimsoidal Systetns

(b) Sketch the ac line cunent waveform J (t) anJ the rectifier outptit vollage waveform Vfi(t).

(c) Determine the ac line current rms magnitutle, fundamentai rms magnitude, and third harmonic rms magnitude. Does this rectifier network conform to the IEC-1000 harmonic current limits?

(d) Determine the power factor, measured at surfaces and S.

The three-phase rectifier of Fig. 16.14 is connected to a balanced 60 Hz Зоас 480 V (rms, line-line) sinusoidal source as shown. All elements are ideal. The inductance L is large, such that the current /(t) is essentially constant, wilh negligible 360 Hz ripple.

BaUmctd

480 V

-JTfSiri-

T f ?

Fig. 16,14 Three-phase rectifier cittuit of IrobltMTi 16.2

(a) Sketch the waveform v{f)

(b) Determine the dc output voltage V,

(e) Sketch the line current waveforms / (0. tfjO), and i(().

(d) Find the Fourier series of ( (f)

(e) Find the distortion factor, displacement factor, power factor, and line current THD.

Harmonic pollution ptdice. In the network of Fig. 16.15, voltage harmonics are observed al the indicated surface. The object of this problem is to decide whether to blame the source or the load for the observed harmonic polluliim. Either the source element or the load element contains a nonhnearity that generates harmonics, while die other element is linear.

Source

Load

Surface S

Fig. 16.15 ,Siitgle-phasc power system of Problems 16.3 to 16.5

(a) Consider first the case where the load is a passive linear impedance Zjis). and hence its phase lies in the range - 90° < ZZj[j(u) < + 90 for aliposiUve to. The source generates harmonics. Express the average power P in the form

where P, is lhe tiveriige power iQinsmilled U) lhe l(j;id by hiirmonit: питЬег п. What tin you ndy Liboiil ihe pokirlti-ен of the Js